Critical Value Calculator (T & Z)

Critical value calculator is a useful tool to measure t value, z value, chi-square value as well as F and R value. Those days are gone when you needed to look in the tables and scan hundreds of values to find a corresponding value for your data.

You can use t critical value calculator to find any type of critical value. With a simple and compelling interface, our t table calculator makes it easy to perform calculations for calculating critical values for any kind of testing.

What is a critical value?

A critical value specifies the margin of error in a distribution graph when analyzing statistics. If a research statistic's absolute value is greater than the critical value, then a statistical sense denies an accepted hypothesis.

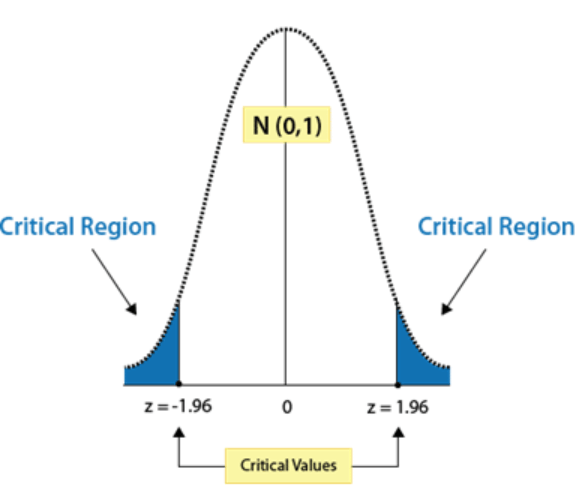
Critical value formula

The critical value formula is the standard equation for the probability.

p = 1 – α/2

Where alpha (α) represents the significance or confidence level, and p is the probability.

In this equation, the alternative hypothesis is considered as alpha. If this hypothesis is true, the null hypothesis is not accepted.

The critical value can be calculated as z score or t score if the distribution of a sampling range is usual or near to normal. You can use our f critical value calculator to find a critical value.

How to find t critical value?

To find the critical value of t, follow the below example.

Example:

The t value is calculated by using the t distribution table. Let's assume degree of freedom as 4, alpha level of 0.025 and conduct a right-tailed test.

In the t distribution table given below, search for the degrees of freedom and its parallel alpha level. The critical value will be found at the intersection of a row and column.

df	α = 0.1	0.05	0.025	0.01	0.005
∞	ta= 1.2816	1.6449	1.96	2.3263	2.5758
1	3.078	6.314	12.706	31.821	63.656
2	1.886	2.920	4.303	6.965	9.925
3	1.638	2.353	3.182	4.541	5.841
4	1.533	2.132	2.776	3.747	4.604
5	1.476	2.015	2.571	3.365	4.032

For the alpha level 0.025, and degrees of freedom 4, the critical value of t will be 2.776.

T critical value = 2.776.

Moreover, you can use our t value calculator to find the t value at one click.

How to find z critical value?

Example:

We will assume significance level (α) of 0.03 to find the z critical value for two tailed test.

Step 1: Divide the significance level by 2.

α/2 = 0.03/2

α/2 = 0.015

Step 2: Calculate (1 - α/2).

1 - α/2 = 1 – 0.015

1 - α/2 = 0.985

Step 3: Look for 0.985 in z table. Add the corresponding value of first row and most left (first) column.

2.1 + 0.07 = 2.17

Z value = ± 2.17

Use z critical value calculator if you are not into prolonged calculations.

What is Chi-Square Value?

One way to show a relationship between two categorical variables is a Chi-square statistic.

The chi-square statistic compares two different variables to determine whether they are related. The numbers are determined by looking at the Chi-square table. Our chi square critical value calculator above eliminates the need to look for values in the tables.